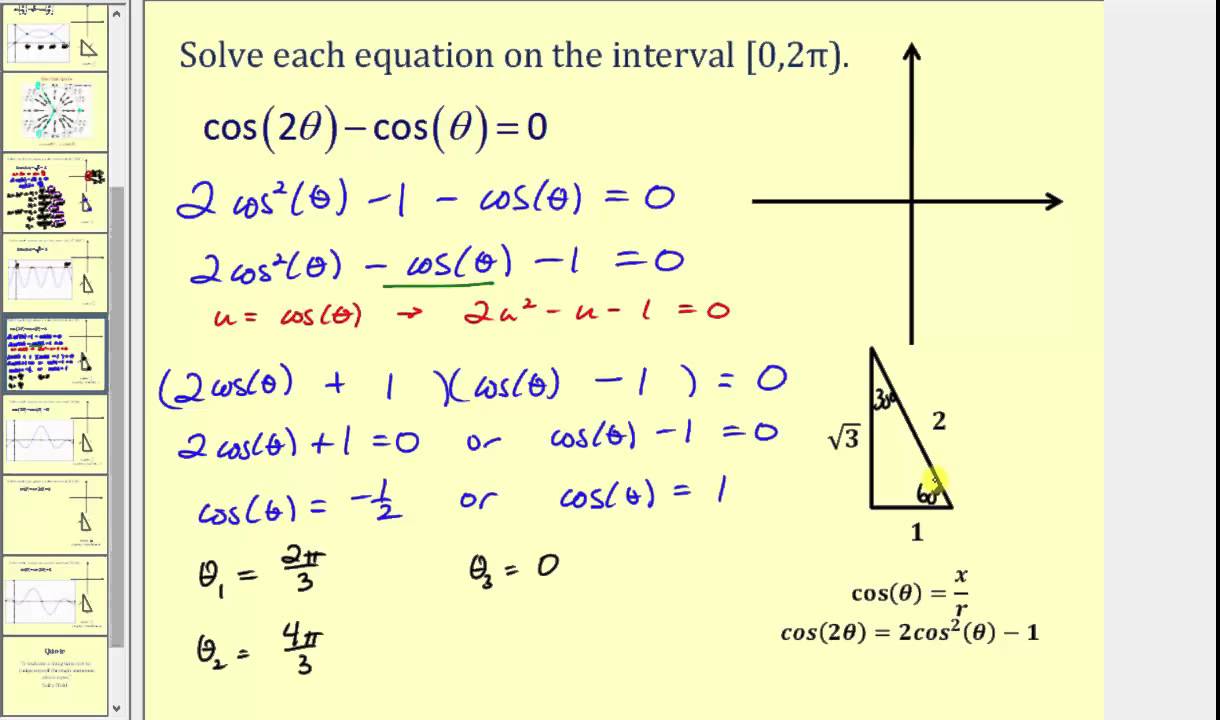
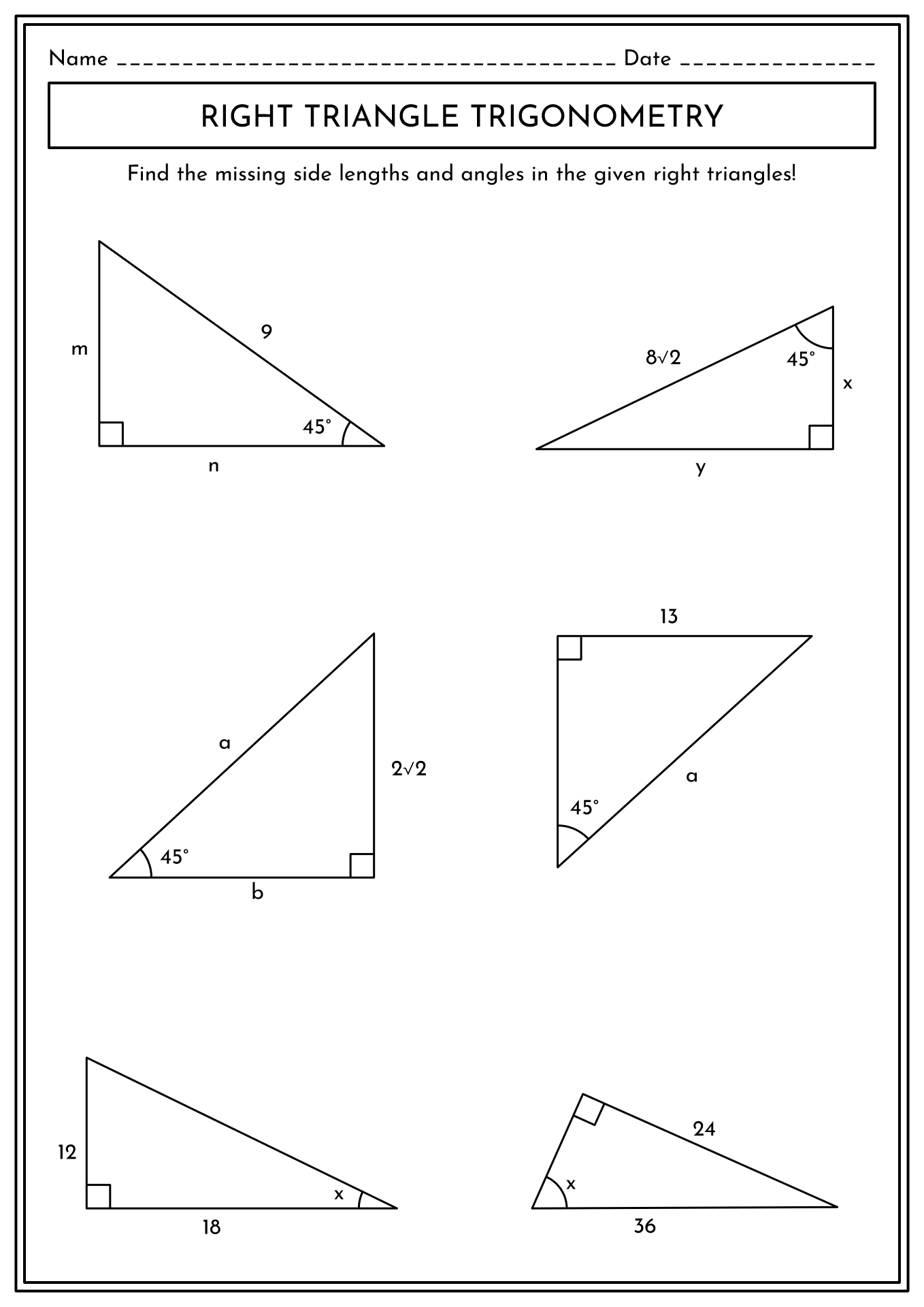
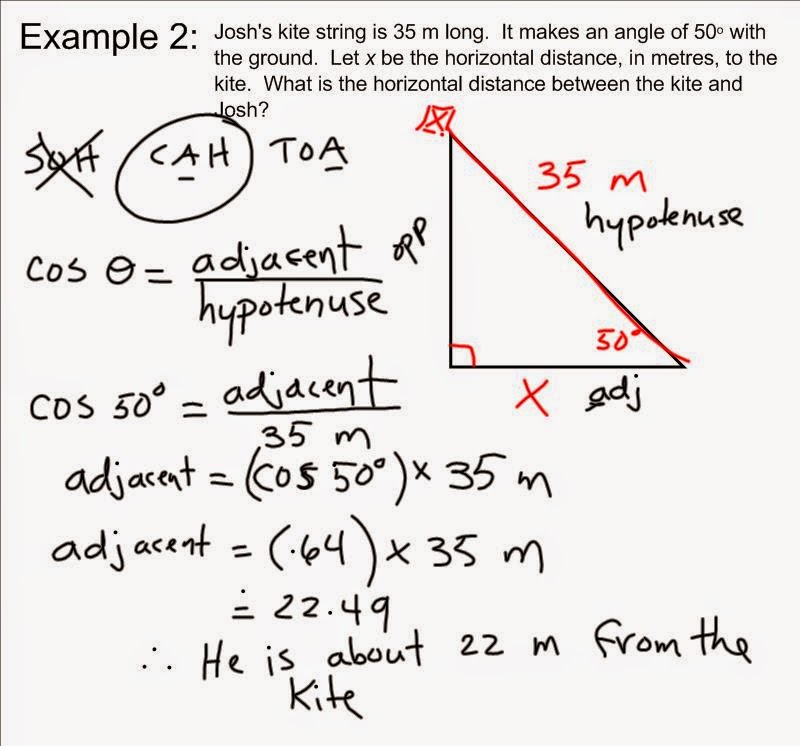
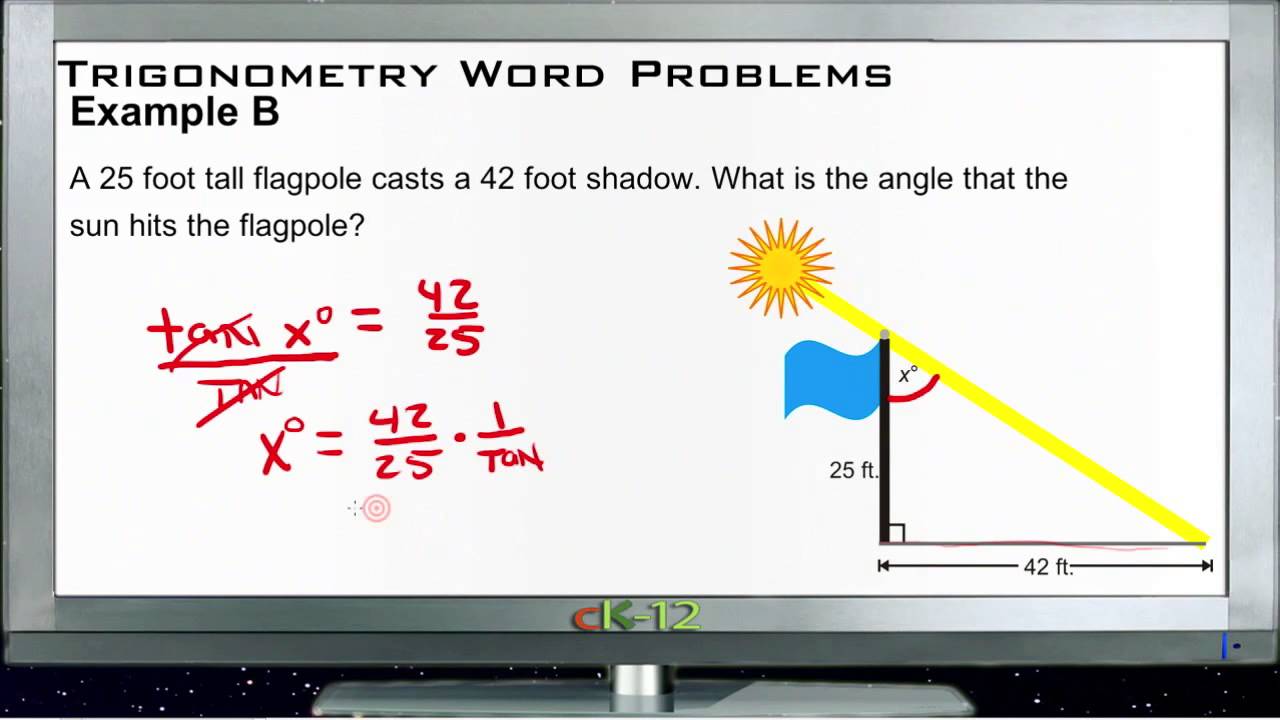
From the towering pyramids of ancient Egypt to the intricate circuitry of modern smartphones, trigonometry has played a silent yet crucial role in shaping our world. It's a branch of mathematics that deals with the relationships between the sides and angles of triangles, offering a powerful toolkit for measurement, calculation, and problem-solving in countless fields.
But what exactly is it about a trigonometry problem that sparks curiosity and, at times, a hint of trepidation? Perhaps it's the elegant dance between geometry and algebra, where abstract concepts like sine, cosine, and tangent come to life within the tangible form of a triangle. Or maybe it's the sheer versatility of trigonometric principles, finding applications in fields as diverse as astronomy, architecture, music, and computer graphics.
The quest to "give me a trigonometry problem" is more than just a request for a mathematical challenge. It's an invitation to explore the rich history and far-reaching implications of this ancient discipline. From the early Babylonian astronomers who used trigonometry to track celestial bodies to the modern-day engineers who rely on it to design bridges and skyscrapers, the study of triangles has been an integral part of human progress.
To truly appreciate the significance of a trigonometry problem, we must delve into its historical roots. The word "trigonometry" itself is derived from the Greek words "trigonon" (triangle) and "metron" (measure), reflecting its origins in the ancient world. Early civilizations like the Egyptians and Babylonians developed rudimentary trigonometric concepts to solve practical problems related to surveying, navigation, and timekeeping.
However, it was the ancient Greeks who formalized trigonometry into a distinct branch of mathematics. Mathematicians like Hipparchus and Ptolemy developed sophisticated trigonometric tables and identities that laid the foundation for future advancements. The development of trigonometry continued through the centuries, with contributions from Indian, Persian, and Arabic mathematicians. It was during the Renaissance that trigonometry found its way into Europe, where it became an essential tool for navigation, astronomy, and cartography.
Advantages and Disadvantages of Solving Trigonometry Problems
| Advantages | Disadvantages |
|---|---|
| Enhances problem-solving skills | Can be abstract and challenging |
| Develops spatial reasoning abilities | Requires a strong foundation in algebra and geometry |
| Applicable in numerous fields | Certain concepts can be difficult to visualize |
The beauty of trigonometry lies not just in its historical significance but also in its practical applications. From calculating the height of a building to determining the distance to a star, trigonometry provides the tools to measure and understand the world around us. It's this blend of theoretical elegance and real-world relevance that makes "give me a trigonometry problem" more than just a classroom exercise; it's a doorway to a universe of possibilities.
Trigonometric Functions And Equations - Trees By Bike
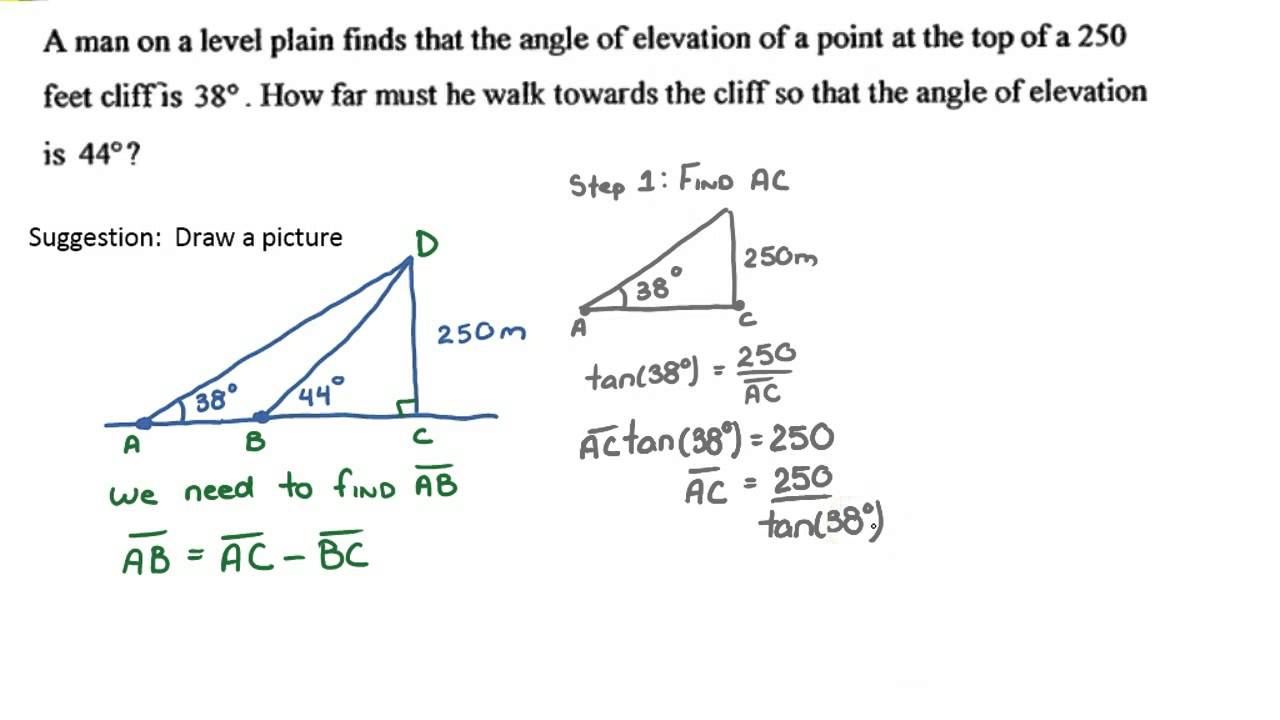
give me a trigonometry problem - Trees By Bike
Trig angle cheat sheet - Trees By Bike
give me a trigonometry problem - Trees By Bike
give me a trigonometry problem - Trees By Bike
give me a trigonometry problem - Trees By Bike
give me a trigonometry problem - Trees By Bike
give me a trigonometry problem - Trees By Bike
give me a trigonometry problem - Trees By Bike
give me a trigonometry problem - Trees By Bike
Trig Word Problems Worksheet - Trees By Bike
give me a trigonometry problem - Trees By Bike
Primary Trigonometric Ratios Worksheet - Trees By Bike
Question Video: Using Right - Trees By Bike